|
YOSHISHIGE OKUNO, SHINRO MASHIKO We propose a rate-costant expression for an excitation-energy transfer between two molecules that is regarded as a nonadiabatic transition between two adiabatic electronic states. It is shown that under weak intermolecular interaction the approximately derived rate constant increases with the increase in pseudo-Coulombic interaction 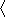  eA eA gB|H| gB|H| gA gA eB eB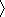 r, and depends on the differences in the potential energies and the potential derivatives of the initial and final electronic states. In addition, the rate constant under strong interaction is show to decrease with increasing pseudo-Coulombic interaction. We show that our expression has the advantage that it can be applied to the excitation-energy transfer under the (not very) weak and the strong coupling limits which cannot be addressed by Forster's theory. r, and depends on the differences in the potential energies and the potential derivatives of the initial and final electronic states. In addition, the rate constant under strong interaction is show to decrease with increasing pseudo-Coulombic interaction. We show that our expression has the advantage that it can be applied to the excitation-energy transfer under the (not very) weak and the strong coupling limits which cannot be addressed by Forster's theory.
|